Hi Stuka,
Plug these into a spreadsheet or mathcad and it will be easy to make adjustments as needed.
Assuming you are burning kerosene and the throat is choked and there is no divergent nozzle your gas properties at the throat will be approximately:
www.grc.nasa.gov/www/k-12/rocket/rktthsum.html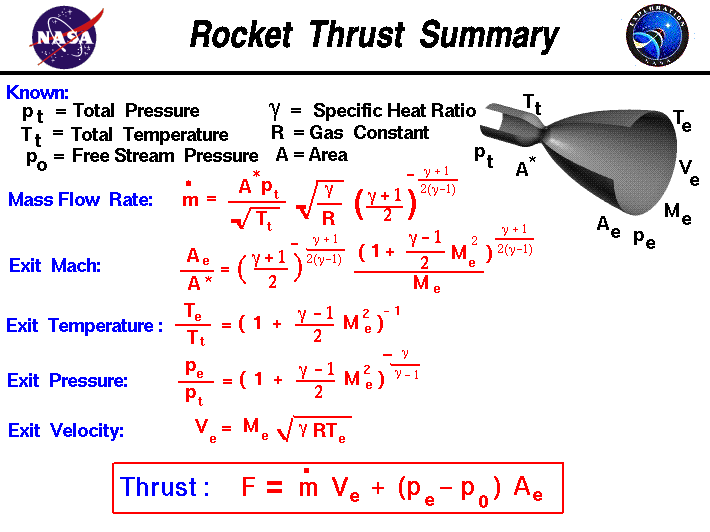
Then for your combustion gas properties, assuming a reasonable combustion gas temperature at full throttle using Gasoline, Kerosene or JP4.
Below is a rough approximation of the combustion gas properties in the chamber and at the nozzle exit.
The theoretical thermodynamic results for gasoline, kerosene and JP4 are pretty much the same when burning this lean.
Calculated using Propep.
44 AIR (DRY AT SEA LEVEL) 977.000 0 0.00001 835N 224O 5AR
552 JP4 (LIQUID TURBOJET FUEL) 23.000 -281 0.02540 17H 9C
THE PROPELLANT DENSITY IS 0.00001 LB/CU-IN OR 0.0003 GM/CC
THE TOTAL PROPELLANT WEIGHT IS 1000.0000 GRAMS
NUMBER OF GRAM ATOMS OF EACH ELEMENT PRESENT IN INGREDIENTS
3.122130 H 1.652893 C 52.698574 N 14.137102 O
0.315560 AR
****************************CHAMBER RESULTS FOLLOW *****************************
T(K) T(F) P(ATM) P(PSI) ENTHALPY ENTROPY CP/CV GAS RT/V
1190. 1683. 3.74 55.00 -6.46 1925.68 1.3035 34.198 0.109
SPECIFIC HEAT (MOLAR) OF GAS AND TOTAL= 8.488 8.456
NUMBER MOLS GAS AND CONDENSED= 34.1982 0.3156
26.34628 N2 4.63263 O2 1.65239 CO2 1.56077 H2O
0.31556 Ar* 0.00545 NO 0.00014 NO2 0.00004 HO
THE MOLECULAR WEIGHT OF THE MIXTURE IS 28.974
****************************EXHAUST RESULTS FOLLOW *****************************
T(K) T(F) P(ATM) P(PSI) ENTHALPY ENTROPY CP/CV GAS RT/V
868. 1381 1.00 14.69 -98.18 1925.68 1.3274 34.198 0.029
SPECIFIC HEAT (MOLAR) OF GAS AND TOTAL= 8.010 7.983
NUMBER MOLS GAS AND CONDENSED= 34.1983 0.3156
26.34897 N2 4.63539 O2 1.65239 CO2 1.56079 H2O
3.16E-01 Ar* 1.83E-04 NO 2.25E-05 NO2
THE MOLECULAR WEIGHT OF THE MIXTURE IS 28.974
**********PERFORMANCE: FROZEN ON FIRST LINE, SHIFTING ON SECOND LINE**********
IMPULSE IS EX
T*
P*
C*
ISP*
OPT-EX
D-ISP
A*M EX-T
89.3
1.3150
1028 2.03
2853
1.0
0.0
1.60916
868.
Notes on output:
1. The gram-atom amounts for each chemical element are based on
the given system weight.
2. The enthalpy has units of kilocalories per system weight and the
entropy has units of calories/K per system weight.
3. GAS identifies the number of moles of gas produced per system
weight. Effective molecular weight is obtained by dividing GAS
into system weight. Note that although non-gases are not included
in this computation this is the proper molecular weight to use in
gas dynamic equations.
4. RT/V = [ R(0.08205 l-atm/mole/K) * T(K) ]/V (system vol. in liters)
5. The damped and undamped speed of sound is calculated assuming
frozen chemical equilibrium conditions. These numbers are equal
if no solids or liquids are present in the plume.
6. The chamber and exhaust composition follow in units of moles per
system weight. If one prefers to obtain partial pressures in
atmospheres, multiply each composition by RT/V. Species are
solid if & follows the specie name and liquid if * follows the
specie name.
7. The first line of the performance results refers to frozen flow,
no chemical reactions, through the nozzle; and the second line
refers to shifting flow, reactions in equilibrium, through the
nozzle. The theoretical exhaust velocity can be found by
multiplying ISP by 9.806 m/sec**2.
8. IS EX is the isentropic exponent such that PV**IS EX = constant
for isentropic flow near the nozzle throat. The values of IS EX
and CP/CV do not agree, because the gas in not perfect.
9. The variables T* and P* are throat temperature (in K) and pressure
(in atmospheres).
10. C* is the characteristic velocity in ft/sec, the nozzle thrust
coefficient, CF = 32.17 * ISP / C*.
11. ISP* is the vacuum impulse to be obtained from a sonic nozzle.
That term is used in air-breathing propulsion work.
12. OPT EX is the ratio of the nozzle exit area to nozzle throat area
at which exit pressure equals ambient pressure.
13. D-ISP is the density ISP.
The first row indicates the combustion temperature (in Kelvin and degrees F), the chamber pressure as specified, the total enthalpy of the mixture (kcal/system mass),
total entropy of the system (cal/K/system mass), CP/CV, which is the ratio of specific heats, GAS (number of gas moles in the mixture), and RT/V (a conversion factor which is not normally used).
Then entering the combustion values into the NASA nozzle equations above you can calculate the velocity based on your design.
In my own spreadsheet I added several other calculations for designing rocket engines but with a little work it can just as easily be adapted to jet propulsion.
Below is a quick example:
SPECIFICATIONS
Thrust 220.0
Viscosity Fu 0.000003
Isp 107.0
L* 2000
Pc 28.000
Ac/At 6.000
O/F 43.48
Pambient 15.000
D Ox 0.001
CP/CV 1.320
D Fuel 0.075
Mol Wt c 28.97
Visc Ox 0.00000254
Tc (F) 1683
PARAMETERS
Wo (pps) 2.284
Ac 44.464
Wf (pps) 0.053
At 7.411
Wt (pps) 2.336
Ae 7.41
Tc (R) 2143
Ae/At 1.0
Tt (R) 1847
Dc 7.524
Pt 15
Dt 3.072
Me 1.0
De 3.072
Pe 14.7
Lc 44.980
Cf 1.258
(L/D)c 0.795
The Mach number at the exit is = Mach 1.0
The C* is the exhaust gas velocity at the nozzle throat = 2853 Feet per Second
If you can take the time to enter the NASA equations into a spread sheet you can make adjustments to fit your specific situation to get the exact numbers you need. Surf around the NASA website for a lot more information on rocket and jet propulsion. It has pretty much everything you need.
I hope this helps. Let me know if you have any questions.
Tony